
Aperiodic Tilings and the Search for the Elusive Einstein
A talk by Dr. Sarmad Abbasi
To find an example of a tiling most of you can look at your bathroom. For mathematicians it is a way to cover the entire (Euclidean) plane with a set of small identical pieces that we call tiles. Here are few ways to do it:
Several lovely questions come to mind immediately. Can we find new and exciting patterns to cover the entire plane? What set of tiles will allow us to cover the entire plane? Kepler, the great genius, tried something spectacular in this direction. He wanted to cover the entire plane in a way that no pattern ever repeats. Such tilings are called aperiodic (a mathematicians way of saying never repeating). His tiling contained a tile that is now called Keplers’ monster!
Later, mathematicians realised that although, his tiling was aperiodic (non-repeating), it was possible to give a repeating pattern using his tiles.
Thus, a deep and interesting question arose: Is it possible to find a set of tiles that is inherently (non-repeating) aperiodic?
Building on the work of Wang, the answer to this question was given affirmatively by Berger in 1966. He produced a set of 20,426 tiles that was inherently (aperiodic) non-repeating. You could use these tiles to cover the entire plane, but you could never get a repeating pattern..
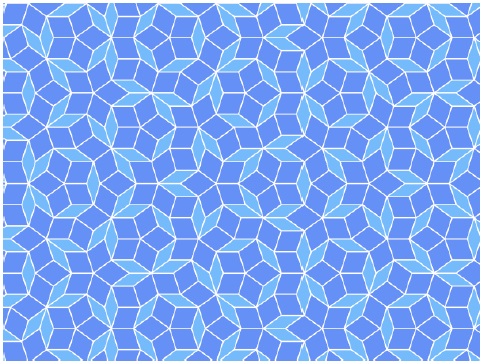
Mathematicians then got to work. They wanted to find smaller and smaller number of tiles that were aperiodic. Roger Penrose, a Nobel prize wining physicist, gave a set of two tiles that had this amazing property.
Then began the final search: Was it possible to find a single aperiodic tile?
Such a tile will be called “ein stien” (A “single stone” in German). Does an einstein exist?
50 years after Penrose’s tiles, mathematicians have found one. This is a recent discovery and certainly a big one.
We will tell the story of this problem and discuss the “Einstein” found recently. We will show how it covers the entire plane.
The lecture will mostly be visual. Using animations and computer graphics. We will also discuss, at the end, a problem that young people can work on.
